When you hear “partial sums algorithm,” you may be a little scared of the word “algorithm.” But really all that means is that it is a step by step procedure. When we teach our students the partial sums algorithm we are really just teaching them steps to use place value to solve addition equations. And this is really powerful for young students because it builds number sense and understanding.

The partial sums algorithm can be a very helpful strategy for 2nd graders learning how to add 2-digit numbers.
Now why is it called partial sums? It’s because we get parts of the answer and then add up those parts to get the final answer.
In this blog post, I’ll give you partial sums examples so you can truly understand this strategy before teaching it to your students. Then I’ll share exactly how I teach it to my 2nd grade students so that each student can find success in adding 2 digit numbers.
But first… If you’re a parent who has a child struggling with math, you’ve got to check out Learner. Learner is an online tutoring platform that sets students up for success. To get started take a short quiz about your child’s needs. Then Learner’s Success Management Team reviews that and matches your child with the perfect tutor. Get your $25 trial here.
I’ve learned that students find success with 2-digit addition and subtraction strategies when you teach them the mental math skills involved and emphasize common mistakes. I have a FREE guide that outlines those for each 2-digit strategy. Grab your copy here: The Ultimate Teaching Guide for 2-Digit Addition & Subtraction Strategies
Partial Sums Examples
Let’s first look at the equation 35+18. We line up the numbers according to place value. The tens numbers should be in line and the ones numbers should be in line. Then we add our tens numbers. 3 tens plus 1 ten is 4 tens. 4 tens is 40. So we write 40 down below. Then we add the ones numbers. 5+8=13. So we write 13 underneath the 40 making sure we still line up according to place value. To get our answer we add 40 and 13 to get 53.
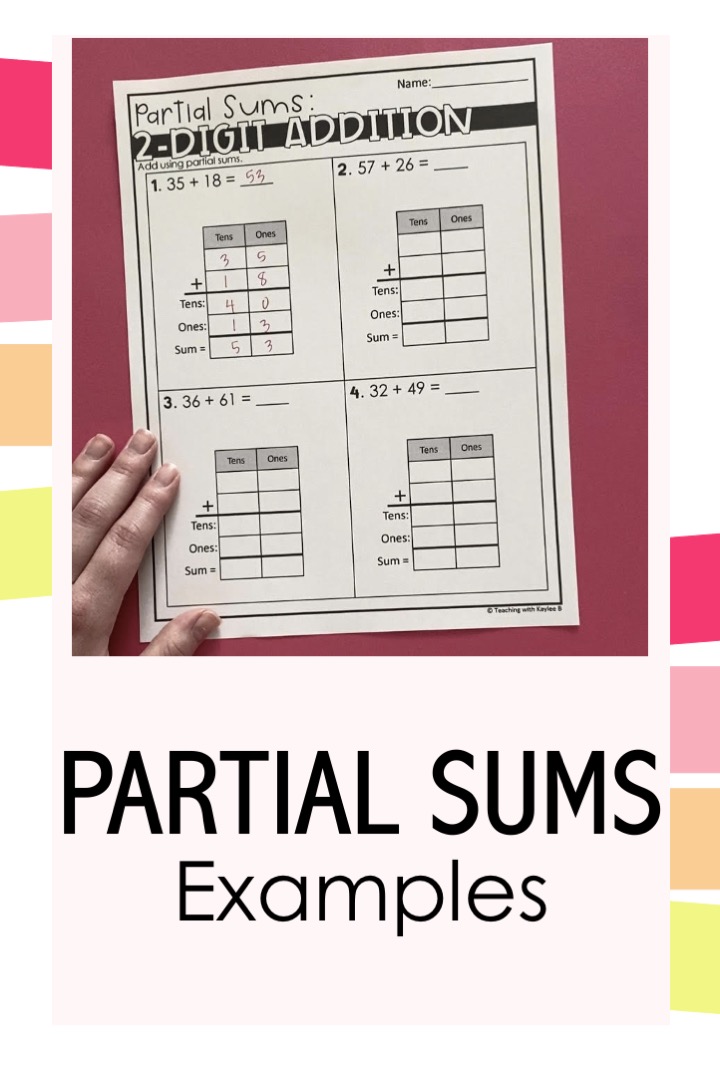
Having the numbers lined up in a chart makes it very easy to focus on place value while using the partial sums algorithm.
Here’s a couple other partial sums examples to look at:
For the equation 46+43 we line those up so the tens are aligned and the ones are aligned. Then add your tens: 4 tens plus 4 tens equals 8 tens and 8 tens equals 80. So we write 80 below. Then add the ones: 6+3=9. We put that 9 below the 80. Make sure that it is lined up according to place value so it would be underneath the 0 in 80. Then add 80+9 to get your answer, 89.
Let me show you another partial sums example with just the numbers and you walk through in your head how it is using place value to add.
28
+14
30
+12
42
From these partial sums examples, you can see how the partial sums algorithm can be very helpful for 2nd grade students. If they know how to add tens and add ones, they are set! They pick up very quickly on the parietal sums strategy and it turns out to be a favorite strategy of many of my 2nd grade students.
So let’s get into how to best teach the partial sums algorithm.
Partial Sums Algorithm
Whenever I am teaching a new strategy to my students I always like to think about what do students need to know beforehand to be successful? And I like to think about what patterns can students pick up on to help them have better success.
Students need to know that for a 2 digit number like 38 that it is made of 3 tens and 8 ones. My 2nd graders also need to know how to add tens together like 3 tens plus 4 tens equals 7 tens and 7 tens equals 70. I find that reviewing these concepts with place value blocks is really helpful. I like to show these blocks up on our front whiteboard. I have magnetic ones that make this really easy. Find the magnetic place value blocks that I use here.
Once we have done that review, I use display pages to easily teach the partial sums strategy. These are like slides that walk me through how to teach the strategy. They make things very visual and have lots of examples for me to work through with my class.
I start off by showing my students examples of the partial sums algorithm where there is a tens and ones chart that helps students line up the numbers according to place value. I also show what these numbers look like with place value blocks. Then I can help students see how we can easily add up the tens, add up the ones, and then add up the tens and the ones.
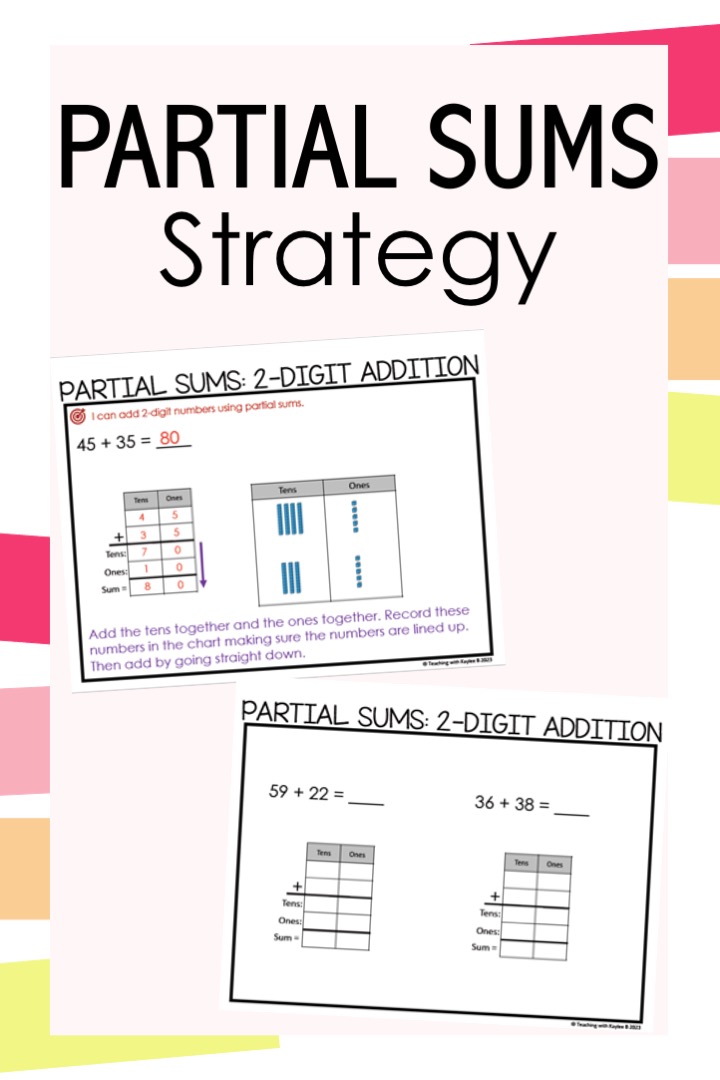
Easily teach each step of the partial sums algorithm with these display pages.
We do a few more examples together as a class with the charts and then I then I get students their own practice with my Partial Sums Worksheets. These have the same charts we used with the problems we did together as a class.
Students solve those problems as I walk around observing and helping students. I take a mental note of any misconceptions and common mistakes I see. Then we come back together and I address those misconceptions and mistakes. We may even do a couple more problems as a class.
Now we won’t always have those charts with us when we need to use the partial sums algorithm. So I teach my students the next step when there isn’t a chart. The display pages line up the numbers and down below there is a “T” to stand for tens and an “O” to stand for ones.

To help create scaffolding as students learn the partial sums algorithm, I like to have my 2nd grade students use worksheets where there is a “T” for Tens and an “O” for Ones.
We follow the same format as before where we solve a couple as a class, then students solve some on their own with my Partial Sums Worksheets, and then help them with misconceptions and common mistakes.
I do this process one more time, but this time only the numbers are lined up. Students have to just remember to line up their numbers according to place value.
These worksheets are so helpful because they support students with each step of the way. They gradually take away support until students can do everything by themselves. Find the partial sums worksheets and display pages I use here.
Partial Sums Strategy
To help my students really master the partial sums strategy, I like to get them some additional practice.
At this point in the lesson, students have been sitting down in their seats for a bit so I want to get them up and moving.
That is why I love to have my students do a scoot activity. I tape up different 2-digit equations around my classroom and give students a recording sheet. They go around the classroom with a partner and solve the problems using the partial sums strategy they just learned. I love having students work with a partner to complete this activity because it gives them support. It also gets them talking about the math.

This partial sums algorithm scoot activity gets students out of the chairs and moving while they practice this strategy.
As I am going around the room monitoring and offering support, I hear my students say things like, “Okay, we start with the tens. We have 5 tens in the first number and 2 tens in the second number. 50+20=70. We need to write 70!” Hearing them talk about the math to each other is one of my favorite parts of the lesson!
After students finish that, I have them check their work with an answer key I set out. They do this with a red pen and then put their page in their Take Home Binder so that their parents can see how they are learning the concept.
Find this partial sums scoot activity to use in your classroom here.
Then to give my students a little more hands-on practice with the partial sums strategy, I have them do a fun puzzle activity with their partner.
This puzzle is a fun partial sums activity that has the initial equations lined up but then students have to find the tens number for underneath, the ones number for underneath, and the sum.

This puzzle activity helps students practice the partial sums algorithm in a fun and engaging way!
I love this puzzle activity because students are so engaged! They love searching through the numbers trying to find the tens answer, the ones answer, and the final answer.
Find this partial sums puzzle activity to use in your classroom here.
When students complete the puzzle activity I have them raise their hands. Then I do a quick check over their work and help them correct any mistakes. They mix up and stack up the pieces and they are ready for the next students.
To really check students’ understanding on the partial sums algorithm, I have students complete an exit ticket individually. They just solve a couple equations and then I check them later on. This helps me see which students might need a little more practice with the strategy in centers.
Find all of my partial sums lesson materials in this resource here.
Partial sums is just one of the strategies I teach for 2-digit addition and subtraction. Find all of my 2-digit addition and subtraction resources in this money-saving bundle here.
Learn more about how to teach the addition strategies in this blog post here. Check out my best tips for teaching the subtraction strategies in this blog post here.
The partial sums strategy is also great for 3-digit addition. Learn how I teach it with 3-digit numbers here.
Find my 3-digit partial sums resources here.
I hope this blog post has been helping in understanding what the parietal sums algorithm is and how to best teach it using 2-digit numbers. It all comes down to using place value to get parts of your answer and then adding those up to get your final answer.
I have found that students do so much better with 2-digit addition and subtraction strategies when they are fluent with their basic facts. I have a free workbook for 1st and 2nd grade students with my best tips for getting your students to math fact mastery. Download your copy of my workbook, The 7 Steps to Ensure Math Fact Fluency, here.
As an Amazon Associate I earn from qualifying purchases.


